What is Linear Equations?
Linear equations are fundamental concepts in mathematics that describe the relationship between two variables. In essence, a linear equation is a mathematical expression that involves variables with an exponent of one. They are called linear equations because they form a straight line when plotted on a graph.
The general form of a linear equation is Ax + By = C, where A, B, and C are constants, and x and y are variables. The constants A and B are called the coefficients of x and y, respectively, while C is the constant term. When graphed on a two-dimensional coordinate plane, the line formed by the linear equation will intersect the x-axis at a point called the x-intercept, and the y-axis at a point called the y-intercept.
Linear equations can be classified into different types based on their number of variables, degree, and form. Here are some of the most common types of linear equations:
The general form of a linear equation is Ax + By = C, where A, B, and C are constants, and x and y are variables. The constants A and B are called the coefficients of x and y, respectively, while C is the constant term. When graphed on a two-dimensional coordinate plane, the line formed by the linear equation will intersect the x-axis at a point called the x-intercept, and the y-axis at a point called the y-intercept.
Linear equations can be classified into different types based on their number of variables, degree, and form. Here are some of the most common types of linear equations:
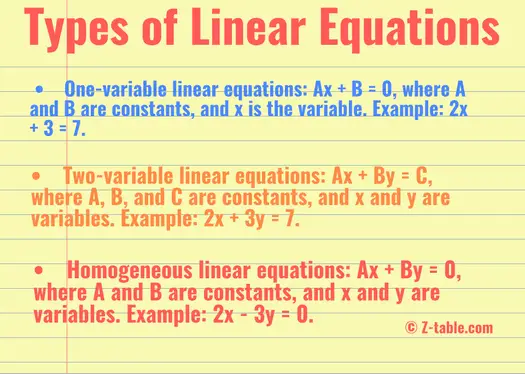
- One-variable linear equations: A one-variable linear equation is an equation that involves only one variable. The general form of a one-variable linear equation is Ax + B = 0, where A and B are constants, and x is the variable. For example, 2x + 3 = 7 is a one-variable linear equation. Read more about One Variable Linear Equations,
- Two-variable linear equations: A two-variable linear equation is an equation that involves two variables. The general form of a two-variable linear equation is Ax + By = C, where A, B, and C are constants, and x and y are variables. For example, 2x + 3y = 7 is a two-variable linear equation.
- Homogeneous linear equations: A homogeneous linear equation is an equation in which all the constants are zero. For example, 2x - 3y = 0 is a homogeneous linear equation.
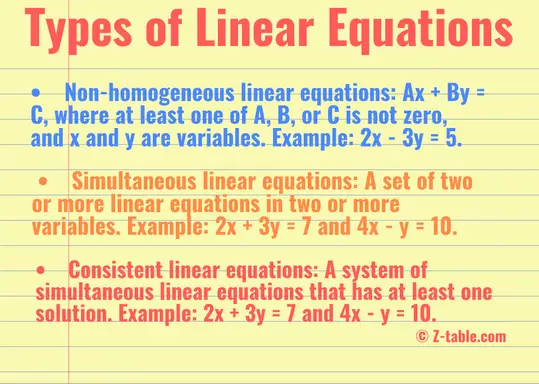
- Non-homogeneous linear equations: A non-homogeneous linear equation is an equation in which at least one constant is not zero. For example, 2x - 3y = 5 is a non-homogeneous linear equation.
- Simultaneous linear equations: A set of two or more linear equations is called a system of simultaneous linear equations. For example, 2x + 3y = 7 and 4x - y = 10 are simultaneous linear equations.
- Consistent linear equations: A system of simultaneous linear equations is consistent if it has at least one solution. In other words, the equations are compatible with each other.
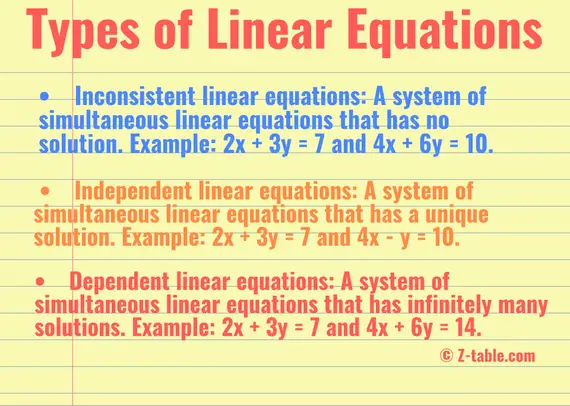
- Inconsistent linear equations: A system of simultaneous linear equations is inconsistent if it has no solution. In other words, the equations are incompatible with each other.
- Independent linear equations: If a system of simultaneous linear equations has a unique solution, it is referred to as an independent system. In other words, the two equations in the system intersect at one point.
- Dependent linear equations: If a system of simultaneous linear equations has infinitely many solutions, it is considered a dependent system. In other words, the two equations in the system represent the same line.
Linear equations are algebraic expressions that describe the relationship between two variables. They can be classified into different types based on their characteristics and properties. Understanding the different types of linear equations is crucial for solving real-world problems and making predictions in various fields. If you need to calculate linear equations check out these resources:
To test you knowledge of linear equations take our tests with answers and explanations:
To test you knowledge of linear equations take our tests with answers and explanations: