Ax=B Linear Equation Calculator
This Ax = B Linear Equation Calculator helps you quickly and easily solve linear equations with a single variable. Simply enter the values for A and B, and the calculator will provide you with the solution, along with a step-by-step explanation of the process. Keep in mind that the equation Ax = B is a linear equation in one variable (x). In this equation, A and B are constants, and x is the variable we want to solve for. This equation represents a straight line when graphed on a coordinate plane. The coefficient 'A' cannot be zero, as it would make the equation undefined.
Solving Ax = B Linear Equations: A Quick Guide
Linear equations are among the most fundamental concepts in mathematics, and they play a vital role in various scientific and engineering disciplines. In this educational post, we will explore the Ax = B linear equation, where A and B are constants, and x is the variable we want to solve for. We'll explain the process of solving such equations, discuss their properties, and provide examples to help you understand the concept better.
What is an Ax = B Linear Equation?
The equation of the form Ax = B, where A and B are fixed real numbers (constants) and x is the variable, is known as a linear equation in one variable. The equation represents a straight line when graphed on a coordinate plane. It's important to note that the coefficient 'A' cannot be zero, as it would make the equation undefined.
Solving Ax = B Linear Equations
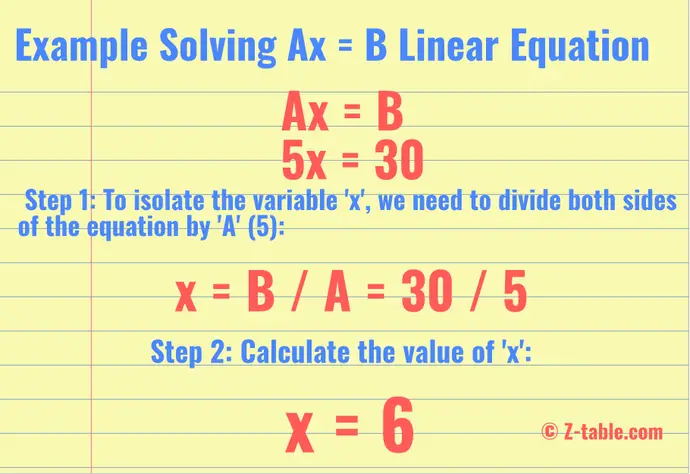
o solve a linear equation in the form Ax = B, follow these steps:
1. Write down the given linear equation: Ax = B
2. To isolate the variable 'x', we need to divide both sides of the equation by 'A': x = B / A
3. Calculate the value of 'x'
Example:
Let's solve the linear equation 3x = 6.
1. Write down the given linear equation: 3x = 6
2. Divide both sides of the equation by 'A' (3): x = 6 / 3
3. Calculate the value of 'x': x = 2
So, the solution to the linear equation 3x = 6 is x = 2.
1. Write down the given linear equation: Ax = B
2. To isolate the variable 'x', we need to divide both sides of the equation by 'A': x = B / A
3. Calculate the value of 'x'
Example:
Let's solve the linear equation 3x = 6.
1. Write down the given linear equation: 3x = 6
2. Divide both sides of the equation by 'A' (3): x = 6 / 3
3. Calculate the value of 'x': x = 2
So, the solution to the linear equation 3x = 6 is x = 2.
Properties of Ax = B Linear Equations
1. A unique solution: For any linear equation in the form Ax = B, there is a unique solution, as long as the coefficient 'A' is not equal to zero. The unique solution is the point where the line intersects the x-axis.
2. Additive property: If two linear equations have the same form (Ax = B), their sum is also a linear equation in the same form. For example, if we have the equations 3x = 6 and 5x = 10, their sum is (3x + 5x) = (6 + 10), which simplifies to 8x = 16, another linear equation.
3. Multiplicative property: If a linear equation in the form Ax = B is multiplied by a constant, the result is still a linear equation in the same form. For example, if we multiply the equation 3x = 6 by 2, we get (2 * 3x) = (2 * 6), which simplifies to 6x = 12, another linear equation.
Solving Ax = B linear equations is an essential skill in mathematics and various other disciplines. By understanding the concept, properties, and solution process, you can effectively tackle problems involving linear equations. The key is to isolate the variable 'x' by dividing both sides of the equation by 'A' and then calculating the value of 'x'. With practice, you'll become proficient at solving linear equations and gain a deeper understanding of the underlying principles.
Check out z-table.com for more mathematics, statistics and unit conversion tools and resources.
2. Additive property: If two linear equations have the same form (Ax = B), their sum is also a linear equation in the same form. For example, if we have the equations 3x = 6 and 5x = 10, their sum is (3x + 5x) = (6 + 10), which simplifies to 8x = 16, another linear equation.
3. Multiplicative property: If a linear equation in the form Ax = B is multiplied by a constant, the result is still a linear equation in the same form. For example, if we multiply the equation 3x = 6 by 2, we get (2 * 3x) = (2 * 6), which simplifies to 6x = 12, another linear equation.
Solving Ax = B linear equations is an essential skill in mathematics and various other disciplines. By understanding the concept, properties, and solution process, you can effectively tackle problems involving linear equations. The key is to isolate the variable 'x' by dividing both sides of the equation by 'A' and then calculating the value of 'x'. With practice, you'll become proficient at solving linear equations and gain a deeper understanding of the underlying principles.
Check out z-table.com for more mathematics, statistics and unit conversion tools and resources.